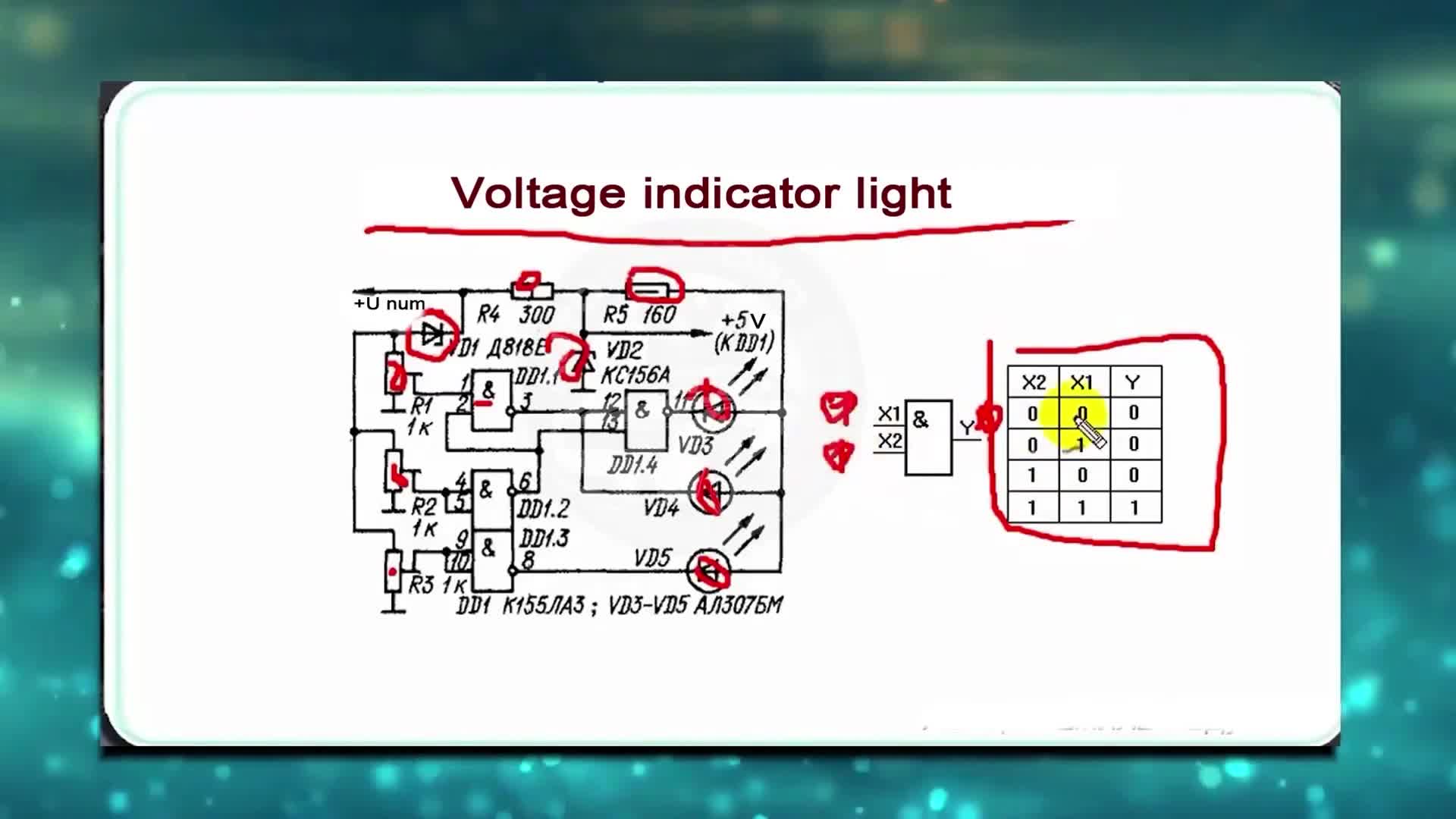
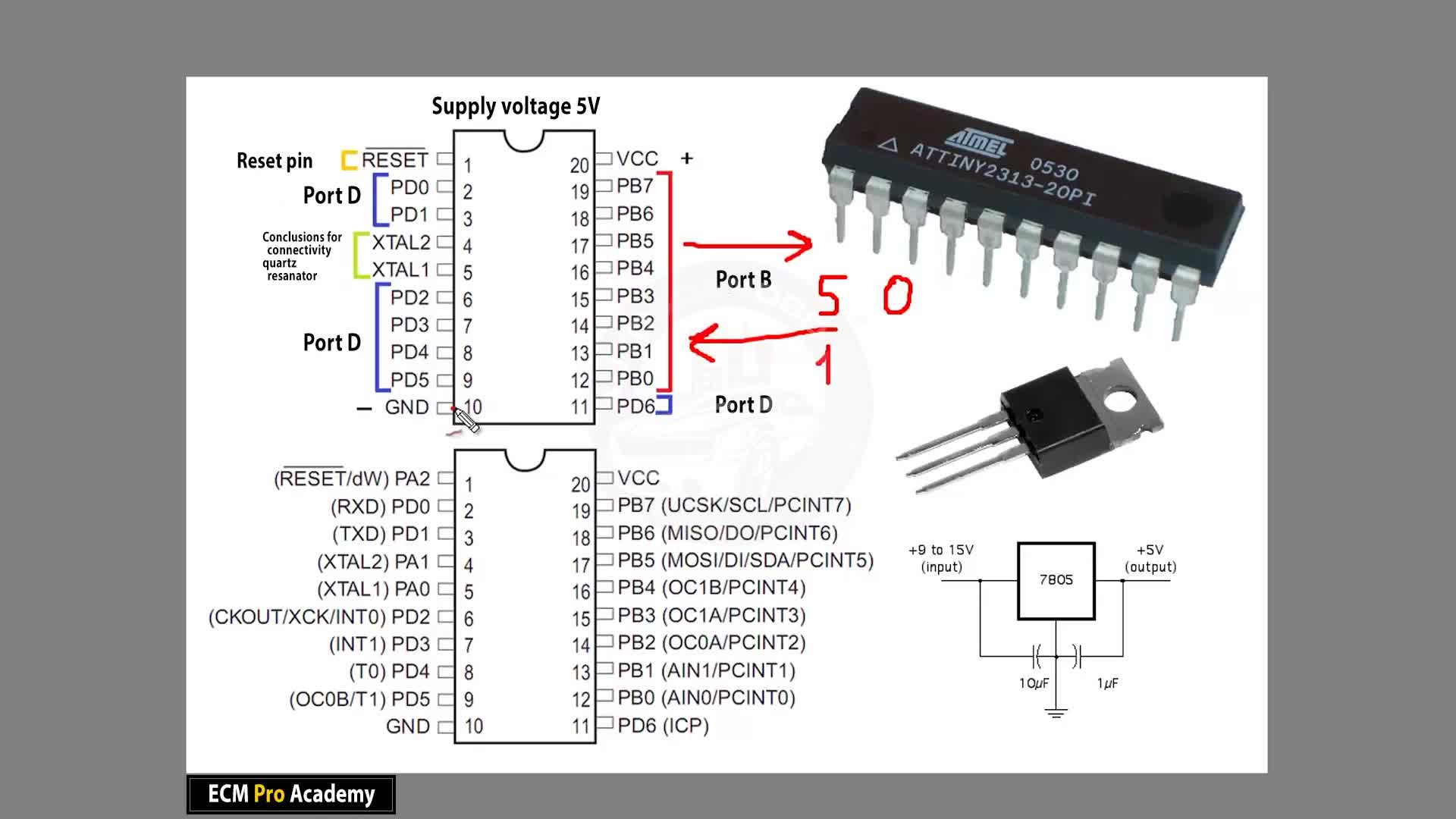
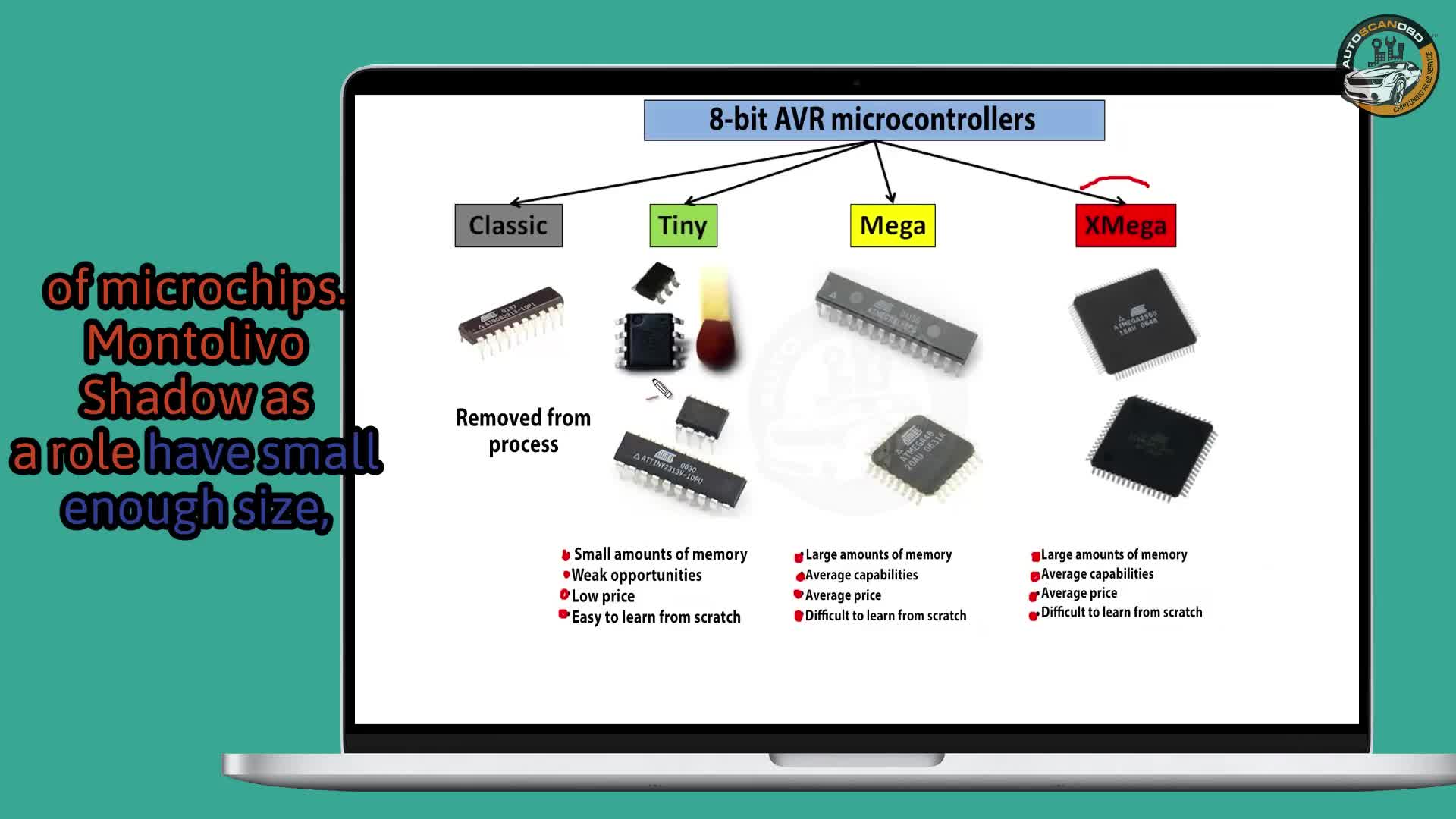
CAPTION
Hi dear student Welcome back to the next explainer video tutorials When we talked about unitary systems of calculus wesaid that they are non positional That is those units with which we counted the number ofmammoths Remember if we swap these sticks with which we counted The result will not change For example heresystems of our thematic there are also positional systems Take the # two 03 If we slapthese numbers around we get 30-203 For example not 302 Either position of numbers has a value Those numericalsystems in which it is impossible to change The position of numbers are called positional In itsturn positional calculus systems are divided into folk and mixed from folk Each position can have avalue the same For example in this position there can be a value from 0-9 Mhm And in thisposition the same calculus system in each position can have the same values Yeah such systems are called folkA calculus system in which in each position there can be the same values For example this time is 21hours 30 minutes in this position the value can be from 0-9 In this position we have avalue from 0-5 minutes and we can have up to 59 16 minutes It is already changing the valueof the next digit and here there is an update Mhm In this position thevalue is also from 0-9 And in this position we have a value only from 0-2 In thesystem of calculation where in different positions different values can be different numbers Mm hmm I mean suchsystems of calculus are called mixed This is just additional information to today's topic It hasno direct relation to In that electronics is used Domestic system of eight river system of 16 riversystem In fact the system of calculus is very and very much mm hmm But we will considersome of them and when we consider these systems you will understand what differences and how they differ Yeah Eachsystem of Calculus has a counter The decimal system has a counter which has 10 teeth in the decimalsystem Hence the name tied system To end The system can only take two values zero and 12 individualsYeah Right Yeah Hence the name System eight River calculus system has also ate values from 0 to 7 Thisis it's counter Hence The Name eight River System Happy And finally 16 river system countered This systemhas 16 values from 0 to 19 river system The 10th value was replaced by the letter a 11thDecember 2013 14 Emmy and 15 EFA total of 16 values to distinguish in what system is written down numbernear this number Usually write an index designating system of calculation the same number in differentsystems can have a different value to distinguish them Mhm mm hmm mm hmm mm Mhm YeahStand such indexes in this theme We with you will consider the real system of calculation Uh huhHere is our tide counter and we will use it to count the number of members Here isthe counter Its value is here when we don't yet have mammoths that is spaceThe counter takes the first value to zero And here we see zero With the appearance of thefirst mammoth the counter takes one step takes the value of one with the appearance ofthe next mammoth The value changes the third tooth And successively we move along the counter the sixthmammoth the seventh the eighth mammoth the night And please note how the value of the counterwill change given that the counter has now ended This last value of the 9th mammoth appears thesurprising because we were moving up the counter 23456789 And with the arrival of the 10th mammoth the counter startscounting from the beginning Yeah That's where the zero appears And where did the 10 mammoths go The mammoths myfriends they went here it's not really 10 It's one and 0 in this position We got azero because the meter ran out of all 10 mammoths that were here They went here as oneAnd then we can see that the weight of this digit turns out to be 10times the weight of this number usually called digits And the digit is counted from right to left Mmhmm mm hmm Mhm mm hmm In this direction it is the junior digit of thesenior digit about this digit If we have a large number there will appear one moredigit respectively This digit will be older than this digit and accordingly older than this one Andthe weight of this digit is 10 times more than this digit and the weightof this digit is 10 times more than this one It is cleared In fact itis not a unit but 10 mammoths that we count 10 mammoths And these 10 mammoths wehave marked as units in this position With the occurrence of 11th mammoth here value changes It is clear withthe occurrence of the second mammoth accordingly value of the younger digit changes because this digit at us countsunits And this digit count stands We and you already spoke about it And what will happenif there are not 12 mammoths but 120 But imagine that each a little bit mammothand mammoths have lined up in 10 rows on 12 pieces So mammoths here are 120 piecesWhen we have counted 10 mammoths on our counter we have begun from 0-9 Here wasa nine with the arrival of the 10th Mama We had a zero in here and one went here whenwe counted 10 more mammoths Another one went here When we counted 10 more Here we got three Sowe got to a nine And with the arrival of 10 more mammoths another nine we got 99 Withthe arrival of another mammoth This unit ended and 10 mammoths went here But already here 19'smammoths were 9x10 Mhm Yeah We had 90 mammoths here We added 10 more mammoths here and thecounter is overflowing Mhm As the counter overflows zero appears here And these mammoths which were in thisdigit They go here as units Since the unit weight in this digit we haveaccumulated 10 values Which way 10 by 10 is 100 respectively This unit value we have in thisdigit we have units Yeah Any value which accepts this digit is to be multiplied by one I it doesnot change What value is such a value The value of this digit is 10 I eachvalue of this digit We should multiply by 10 Uh huh But since in this digit we havetheir value is 100 accordingly When this digit overflows 10 units with a weight of 10 itpasses here and this unit has a weight of 100 So this value in thisdigit is a unit Mm hmm mm hmm In this digit on 10 in this digit of 100If we will have even 10 times more accordingly we will have one more digit and the weight of thisdigit will be 1000 It is clear from a school mathematics course we know that 10 of zerodegrees is equal to unit 10 in the first degree and is equal to 10 10 squared 110 The thirddegree 1000 Mhm Yeah if who has forgotten this material I will remind that the degreeshows how many times the basis to multiply on itself As here stands double in thesquare that this basis to multiply on two times Yeah Yeah Mhm Here they are two basis tomultiply each other Hence the value of 100 Here we have the power of threeSo 10 multiply each other three times 1 10-3/10 multiply each other and their value isthis 10 We can represent as 10 to the first power And here this oneweekend represent as 10 to the zero power We can use this table to write the digit value ofthis number Let's take another number as an example To make it clearer let's take the number 630 Wewrite the value of this number and then translate it We already told you that the lowestdigit value of this number is multiplied by 10 zero degrees Here we take the lowest digit ofthe value of zero We multiply by 10 to 0 degrees Here we take the next digit of thevalue from the next digit three Here we multiply it by 10 to the first powerYeah Three multiplied by 10 to the first power We take the next power of six and multiply it byfour then multiply by 10 to the third degree 0123 days after decomposing by calculate the value of the numberwe can see this combination of numbers has the same form but in another system ofcalculations the value of this number will be quite different Uh huh mm Here is just acoincidence And what do we do if we have one more digit how to change thisformula Well as usual we take this digit value Put a plus take the value multiplied by 10 Andrespectively we will have 01234 the fourth degree If there will be one more value say nine plus 9/10 degreecounting from zero then it is zero digits first digit second digit third digit 4th 5th digit YeahYeah Mhm mm hmm respectively And the degree of zero digit actions zero degree first digit and so onThis is the representation the value of this number in the decimal system Thanks for watching
CAPTION TRANSLATED TO
HINDI LANGUAGE
हाय प्रिय छात्र अगले व्याख्याता वीडियो ट्यूटोरियल में आपका स्वागत है जब हमने कैलकुलस की एकात्मक प्रणाली के बारे में बात की थीने कहा कि वे गैर स्थितीय हैं यानी वे इकाइयाँ हैं जिनके साथ हमने की संख्या गिन ली हैमैमथ याद रखें अगर हम इन स्टिक्स की अदला-बदली करें जिनसे हमने गिनती की थी तो परिणाम नहीं बदलेगा उदाहरण के लिए यहाँहमारे विषयगत प्रणालियों में स्थितीय प्रणालियाँ भी हैं # दो 03 यदि हम थप्पड़ मारते हैंइन नंबरों के आस-पास हमें 30-203 मिलते हैं उदाहरण के लिए 302 नहीं संख्याओं की किसी भी स्थिति का मान होता है वे संख्यात्मकजिन प्रणालियों में संख्याओं की स्थिति को बदलना असंभव है, उन्हें स्थितीय कहा जाता हैटर्न पोजिशनल कैलकुलस सिस्टम लोक में विभाजित होते हैं और लोक से मिश्रित होते हैं प्रत्येक स्थिति में एक हो सकता हैमान समान उदाहरण के लिए इस स्थिति में 0-9 Mhm से मान हो सकता है और इसमेंस्थिति समान कलन प्रणाली प्रत्येक स्थिति में समान मान हो सकते हैं हाँ ऐसी प्रणालियों को लोक कहा जाता हैएक कलन प्रणाली जिसमें प्रत्येक स्थिति में समान मान हो सकते हैं उदाहरण के लिए यह समय 21 . हैघंटे 30 मिनट इस स्थिति में मान 0-9 से हो सकता है इस स्थिति में हमारे पास aअगले अंक का और यहाँ एक अद्यतन है Mhm इस स्थिति मेंमान भी 0-9 से है और इस स्थिति में हमारे पास 0-2 से ही मान होता हैगणना की प्रणाली जहां अलग-अलग स्थितियों में अलग-अलग मान अलग-अलग संख्याएं हो सकते हैं एमएम हम्म मेरा मतलब है किकलन की प्रणालियों को मिश्रित कहा जाता है यह आज के विषय के लिए केवल अतिरिक्त जानकारी हैउस इलेक्ट्रॉनिक्स से कोई सीधा संबंध नहीं है 16 नदी की आठ नदी प्रणाली की घरेलू प्रणाली का उपयोग किया जाता हैप्रणाली वास्तव में कलन की प्रणाली बहुत और बहुत अधिक मिमी हम्म है लेकिन हम विचार करेंगेउनमें से कुछ और जब हम इन प्रणालियों पर विचार करते हैं तो आप समझेंगे कि क्या अंतर हैं और वे कैसे भिन्न हैं हाँ प्रत्येककैलकुलस की प्रणाली में एक काउंटर होता है दशमलव प्रणाली में एक काउंटर होता है जिसमें दशमलव में 10 दांत होते हैंसिस्टम इसलिए नाम बंधा हुआ सिस्टम समाप्त करने के लिए सिस्टम केवल दो मान शून्य और 12 व्यक्ति ले सकता हैहाँ ठीक हाँ इसलिए नाम सिस्टम आठ रिवर कैलकुलस सिस्टम ने भी 0 से 7 तक के मान खा लिए हैंक्या यह काउंटर है इसलिए नाम आठ नदी प्रणाली खुश और अंत में 16 नदी प्रणाली ने इस प्रणाली का मुकाबला कियाइसमें 0 से 19 नदी प्रणाली के 16 मान हैं। 10वें मान को 11वें अक्षर से बदल दिया गया हैदिसंबर 2013 14 एमी और 15 ईएफए कुल 16 मूल्यों में अंतर करने के लिए किस प्रणाली में संख्या लिखी गई हैइस संख्या के पास आमतौर पर एक ही संख्या की गणना के लिए एक सूचकांक नामित प्रणाली लिखेंसिस्टम में उन्हें अलग करने के लिए एक अलग मूल्य हो सकता है एमएचएम मिमी हम्म मिमी हम्म मिमी एमएच हाँइस विषय में ऐसे सूचकांक खड़े करें हम आपके साथ गणना की वास्तविक प्रणाली पर विचार करेंगे उह हुहयहाँ हमारा ज्वार काउंटर है और हम इसका उपयोग सदस्यों की संख्या गिनने के लिए करेंगे यहाँ हैकाउंटर इसका मूल्य यहाँ है जब हमारे पास अभी तक अंतरिक्ष नहीं हैकाउंटर पहले मान को शून्य पर ले जाता है और यहां हमें शून्य दिखाई देता हैपहला विशाल काउंटर एक कदम उठाता है, की उपस्थिति के साथ एक का मूल्य लेता हैअगला मैमथ मान तीसरे दांत को बदल देता है और क्रमिक रूप से हम काउंटर के साथ छठे की ओर बढ़ते हैंमैमथ सातवें आठवें विशाल रात और कृपया ध्यान दें कि काउंटर का मूल्य कैसा हैयह देखते हुए बदल जाएगा कि काउंटर अब समाप्त हो गया है 9वें विशाल का यह अंतिम मान प्रकट होता हैआश्चर्यजनक है क्योंकि हम काउंटर 23456789 ऊपर जा रहे थे और 10वें विशाल के आगमन के साथ काउंटर शुरू हो गयाशुरू से गिनते हुए हाँ जीरो वहीं दिखाई देता है और 10 मैमथ कहाँ गए मैमथ मायदोस्तों वे यहाँ गए थे यह वास्तव में 10 नहीं है यह एक है और 0 इस स्थिति में हमें एक मिला हैशून्य क्योंकि मीटर सभी 10 मैमथ से बाहर चला गया जो यहाँ थे वे यहाँ एक के रूप में गए थेऔर फिर हम देख सकते हैं कि इस अंक का भार 10 . हो गया हैइस संख्या के वजन का गुणा जिसे आमतौर पर अंक कहा जाता है और अंक को दाएं से बाएं Mm . में गिना जाता हैहम्म मिमी हम्म मम मिमी हम्म इस दिशा में यह का कनिष्ठ अंक हैइस अंक के बारे में वरिष्ठ अंक यदि हमारे पास बड़ी संख्या है तो एक और दिखाई देगाअंक क्रमशः यह अंक इस अंक से पुराना होगा और तदनुसार इस अंक से भी पुराना होगाइस अंक का भार इस अंक और भार से 10 गुना अधिक हैइस अंक का इस अंक से 10 गुना अधिक हैएक इकाई नहीं बल्कि 10 मैमथ हैं जिन्हें हम 10 मैमथ गिनते हैं और ये 10 मैमथ हमइस स्थिति में इकाइयों के रूप में चिह्नित किया है 11 वीं विशाल की घटना के साथ यहां मूल्य परिवर्तन यह स्पष्ट हैदूसरे मैमथ की घटना के अनुसार छोटे अंक का मूल्य बदल जाता है क्योंकि यह अंक हमारे लिए मायने रखता हैइकाइयाँ और यह अंकों की गिनती खड़ी है हम और आप पहले ही इसके बारे में बात कर चुके हैं और क्या होगाअगर वहाँ 12 विशाल नहीं बल्कि 120 हैं लेकिन कल्पना कीजिए कि प्रत्येक थोड़ा विशाल हैऔर मैमथ को 10 पंक्तियों में 12 टुकड़ों में पंक्तिबद्ध किया गया है तो मैमथ यहाँ 120 टुकड़े हैंजब हमने अपने काउंटर पर 10 मैमथ गिन लिए तो हमने 0-9 से शुरू कर दिया यहाँ थादसवीं माँ के आगमन के साथ एक नौ हमारे यहाँ एक शून्य था और एक यहाँ गया था जबहमने 10 और मैमथ गिने एक और यहाँ गया जब हमने 10 और गिने तो यहाँ हमें तीन मिलेहम एक नौ पर पहुंच गए और 10 और मैमथ के आने से एक और नौ हमें मिल गए 99 के साथएक और विशाल का आगमन यह इकाई समाप्त हो गई और 10 विशाल यहां चले गए लेकिन यहां पहले से ही 19 केमैमथ 9x10 एमएचएम थे हां हमारे यहां 90 मैमथ थे हमने यहां 10 और मैमथ जोड़े औरकाउंटर ओवरफ्लो हो रहा है महम जैसे ही काउंटर ओवरफ्लो होता है जीरो यहां दिखाई देता है और ये मैमथ जो इसमें थेअंक वे यहां इकाइयों के रूप में जाते हैं क्योंकि इस अंक में इकाई भार हमारे पास हैसंचित 10 मान जिस तरह से 10 बटा 10 क्रमशः 100 है यह इकाई मूल्य हमारे पास हैअंक हमारे पास इकाइयाँ हैं हाँ कोई भी मान जो इस अंक को स्वीकार करता है उसे एक से गुणा किया जाता है Iनहीं बदलें ऐसा कौन सा मान है इस अंक का मान 10 है I प्रत्येकइस अंक का मान हमें 10 से गुणा करना चाहिए, लेकिन चूंकि इस अंक में हमारे पास हैउनका मान 100 तदनुसार होता है जब यह अंक 10 इकाइयों के वजन के साथ 10 इकाइयों से अधिक हो जाता हैयहाँ से गुजरता है और इस इकाई का भार 100 है तो इसमें यह मान हैअंक एक इकाई है मिमी हम्म मिमी हम्म इस अंक में 10 पर 100 . के इस अंक मेंयदि हमारे पास तदनुसार 10 गुना अधिक होगा तो हमारे पास एक और अंक होगा और इसका वजन होगाअंक 1000 होगा यह एक स्कूल गणित पाठ्यक्रम से स्पष्ट है कि हम जानते हैं कि शून्य का 10डिग्री पहली डिग्री में इकाई 10 के बराबर है और 10 10 वर्ग 110 के बराबर है तीसराडिग्री 1000 महम हाँ अगर इस सामग्री को कौन भूल गया है तो मैं याद दिला दूँगा कि डिग्रीदिखाता है कि आधार को कितनी बार अपने आप गुणा करना है जैसा कि यहाँ पर दोगुना हैवर्ग है कि इस आधार को दो गुणा करने के लिए हाँ हाँ महम यहाँ वे दो आधार हैंएक दूसरे को गुणा करें इसलिए 100 का मान यहां हमारे पास तीन की शक्ति हैअतः 10 एक दूसरे को तीन गुना गुणा करें 1 10-3/10 एक दूसरे को गुणा करें और उनका मान हैयह 10 हम पहली शक्ति के लिए 10 के रूप में प्रतिनिधित्व कर सकते हैं और यहाँ यह हैसप्ताहांत 10 से शून्य शक्ति के रूप में प्रतिनिधित्व करते हैं हम इस तालिका का उपयोग अंकों का मान लिखने के लिए कर सकते हैंयह संख्या आइए एक और संख्या को उदाहरण के रूप में लेते हैं इसे स्पष्ट करने के लिए आइए संख्या 630 लेते हैं हमइस संख्या का मूल्य लिखें और फिर इसका अनुवाद करें हमने आपको पहले ही बताया था कि सबसे कमइस संख्या के अंकों के मान को 10 शून्य डिग्री से गुणा किया जाता है। यहां हम का सबसे छोटा अंक लेते हैंशून्य का मान हम 10 से 0 डिग्री से गुणा करते हैं यहां हम का अगला अंक लेते हैंअगले अंक तीन से मान यहाँ हम इसे 10 से पहली शक्ति से गुणा करते हैंहाँ तीन को पहली घात से 10 से गुणा किया जाता है हम छः की अगली घात लेते हैं और इसे गुणा करते हैंचार फिर संख्या के मान की गणना करके विघटित होने के 0123 दिनों के बाद 10 से तीसरी डिग्री तक गुणा करेंहम देख सकते हैं कि संख्याओं के इस संयोजन का एक ही रूप है लेकिन एक अन्य प्रणाली मेंगणना इस संख्या का मूल्य काफी अलग होगा उह हुह मिमी यहाँ सिर्फ एक हैसंयोग और हम क्या करें यदि हमारे पास एक और अंक है तो इसे कैसे बदलेंफ़ॉर्मूला हमेशा की तरह हम इस अंक का मान लेते हैं, एक जोड़ लगाएं और मान को 10 से गुणा करें औरक्रमशः हमारे पास 01234 चौथी डिग्री होगी यदि एक और मान होगा नौ प्लस 9/10 डिग्रीशून्य से गिनने पर यह शून्य अंक होता है पहला अंक दूसरा अंक तीसरा अंक चौथा पांचवां अंक हाँहाँ महम मिमी हम्म क्रमशः और शून्य अंकों की क्रियाओं की डिग्री शून्य डिग्री पहले अंक और इसी तरहयह दशमलव प्रणाली में इस संख्या के मूल्य का प्रतिनिधित्व है देखने के लिए धन्यवाद
CAPTION TRANSLATED TO
ARABIC LANGUAGE
مرحبًا عزيزي الطالب مرحبًا بك مرة أخرى في دروس الفيديو التوضيحية التالية عندما تحدثنا عن الأنظمة الوحدوية لحساب التفاضل والتكاملقالوا إنهم غير موضعيين أي تلك الوحدات التي حسبنا عددهاتذكر أنه إذا قمنا بتبديل هذه العصي التي حسبناها فلن تتغير النتيجة على سبيل المثال هناالأنظمة الموضوعية لدينا هناك أيضًا أنظمة تحديد المواقع. خذ الرقمين 03 إذا صفعناهذه الأرقام حولنا نحصل على 30-203 على سبيل المثال ليس 302 أي من موضع الأرقام له قيمة تلك العدديةالأنظمة التي يستحيل فيها تغيير موضع الأعداد تسمى الموضعية بداخلهابدوره نظم حساب التفاضل والتكامل مقسمة إلى قوم ومختلطة من قوم كل موقف يمكن أن يكونالقيمة نفسها على سبيل المثال في هذا الموضع يمكن أن يكون هناك قيمة من 0-9 Mhm وفي هذاوضع نفس نظام حساب التفاضل والتكامل في كل موقف يمكن أن يكون لها نفس القيم نعم تسمى هذه الأنظمة قومنظام حساب التفاضل والتكامل حيث يمكن أن توجد نفس القيم في كل مركز على سبيل المثال هذه المرة هي 21ساعة 30 دقيقة في هذا الموضع يمكن أن تكون القيمة من 0-9 في هذا الموضع لديناالقيمة من 0-5 دقائق ويمكن أن يكون لدينا 59 16 دقيقة وهي تغير القيمة بالفعلمن الرقم التالي وهنا يوجد تحديث Mhm في هذا الموضعالقيمة أيضًا من 0-9 وفي هذا الموضع لدينا قيمة فقط من 0-2 فينظام الحساب حيث يمكن أن تكون القيم المختلفة في المواقف المختلفة أرقامًا مختلفة مم همم أعني هذاتسمى أنظمة التفاضل والتكامل مختلطة. هذه مجرد معلومات إضافية لموضوع اليوملا توجد علاقة مباشرة في هذا الإلكترونيات يستخدم النظام المحلي لثمانية أنظمة نهرية من 16 نهرًاالنظام في الواقع نظام حساب التفاضل والتكامل كبير جدًا جدًا مم همم لكننا سننظر فيهبعضها وعندما نفكر في هذه الأنظمة سوف تفهم ما هي الاختلافات وكيف تختلف نعم كل منهمانظام حساب التفاضل والتكامل له عداد النظام العشري له عداد به 10 أسنان في الكسر العشريالنظام ومن هنا اسم نظام ربط حتى النهاية يمكن للنظام أن يأخذ قيمتين فقط صفر و 12 فردًانعم ، صحيح نعم ، ومن هنا جاء اسم نظام حساب التفاضل والتكامل System eight الذي تناول أيضًا قيمًا من 0 إلى 7 هذاهو العداد ومن هنا جاء اسم نظام النهر الثامن سعيد وأخيراً واجه نظام النهر 16 هذا النظاميحتوي على 16 قيمة من 0 إلى 19 نظام نهري. تم استبدال القيمة العاشرة بالحرف أ 11ديسمبر 2013 14 Emmy و 15 EFA مجموعها 16 قيمة للتمييز في أي نظام مكتوب بالرقمبالقرب من هذا الرقم عادةً ما تكتب نظامًا لتعيين الفهرس لحساب نفس الرقم بشكل مختلفيمكن أن يكون للأنظمة قيمة مختلفة لتمييزها Mhm mm hmm mm hmm mm Mhm نعمتقف مثل هذه الفهارس في هذا الموضوع ونحن معكم سننظر في نظام الحساب الحقيقي أه هاهها هو عداد المد والجزر الخاص بنا وسنستخدمه لحساب عدد الأعضاء هناالعداد تكون قيمته هنا عندما لا يكون لدينا بعد الماموث وهو الفضاءيأخذ العداد القيمة الأولى إلى الصفر وهنا نرى الصفر مع ظهورالماموث الأول يأخذ العداد خطوة واحدة يأخذ قيمة واحدة مع مظهرالماموث التالي تتغير القيمة للسن الثالث ونتحرك على التوالي على طول العداد السادسالماموث السابع الماموث الثامن الليل ويرجى ملاحظة قيمة العدادسوف يتغير نظرًا لأن العداد قد انتهى الآن. تظهر القيمة الأخيرة للماموث التاسعالماموث العاشر ويرجى ملاحظة أنه في هذا الرقم لدينا مرة أخرى صفر وهذا ليس كذلكمفاجأة لأننا كنا نتحرك لأعلى العداد 23456789 ومع وصول الماموث العاشر يبدأ العدادالعد من البداية نعم هذا هو المكان الذي يظهر فيه الصفر وأين ذهب الماموث العشرة؟الأصدقاء الذين ذهبوا إلى هنا ليس 10 حقًا ، إنه واحد و 0 في هذا المنصبصفر لأن العداد نفد من كل الماموث العشرة التي كانت هنا. لقد ذهبوا هنا كواحدثم نلاحظ أن وزن هذا الرقم اتضح أنه 10أضعاف وزن هذا الرقم عادةً ما يسمى بالأرقام ويتم حساب الرقم من اليمين إلى اليسار ممhmm mm hmm Mhm mm hmm في هذا الاتجاه هو الرقم الأصغر منرقم كبير حول هذا الرقم إذا كان لدينا عدد كبير فسيظهر رقم واحد آخررقم على التوالي سيكون هذا الرقم أقدم من هذا الرقم وبالتالي أقدم من هذا الرقم ووزن هذا الرقم 10 مرات أكثر من هذا الرقم والوزنمن هذا الرقم أكبر بعشر مرات من هذا الرقم تم مسحه في الواقعليست وحدة ولكن 10 ماموث نحصي 10 ماموث وهذه العشرة ماموث نحنتم وضع علامة كوحدات في هذا الموضع مع حدوث الماموث الحادي عشر هنا تتغير القيمة من الواضح معحدوث الماموث الثاني وفقًا لذلك تتغير قيمة الرقم الأصغر لأن هذا الرقم مهم عندناالوحدات وهذا العدد من الأرقام يقف نحن وأنت تحدثنا عنها بالفعل وماذا سيحدثإذا لم يكن هناك 12 من الماموث ولكن 120 ولكن تخيل أن كل واحد منهم عملاق قليلاًوالماموث اصطفوا في 10 صفوف على 12 قطعة ، لذا فإن الماموث هنا 120 قطعةعندما أحصينا 10 من الماموث على منضدنا ، بدأنا من 0-9 هناتسعة مع وصول الأم العاشرة كان لدينا صفر هنا وواحد ذهب هنا عندماأحصينا 10 ماموث أخرى وذهب آخر هنا عندما أحصينا 10 أخرى هنا حصلنا على ثلاثةوصلنا إلى تسعة ومع وصول 10 ماموث أخرى تسعة أخرى حصلنا على 99 معوصول عملاق آخر انتهت هذه الوحدة وذهب 10 ماموث هنا ولكن هنا بالفعل 19كانت أعداد الماموث 9 × 10 ميجا هرتز نعم كان لدينا 90 من الماموث هنا أضفنا 10 من الماموث هنا والعداد يفيض Mhm حيث أن العداد يفيض بالصفر يظهر هنا وهذه الماموث التي كانت في هذاdigit يذهبون هنا كوحدات منذ أن أصبح لدينا وزن الوحدة في هذا الرقمقيم متراكمة 10 أي اتجاه 10 في 10 يساوي 100 على التوالي قيمة الوحدة هذه لدينا في هذاالرقم لدينا وحدات نعم أي قيمة تقبل هذا الرقم يجب ضربها بواحدلا يتغير ما هي القيمة مثل هذه القيمة قيمة هذا الرقم هي 10 أنا لكل منهماقيمة هذا الرقم يجب أن نضرب في 10 آه هاه ولكن بما أننا في هذا الرقم لديناقيمتها 100 وفقًا لذلك عندما يتجاوز هذا الرقم 10 وحدات بوزن 10يمر هنا ويبلغ وزن هذه الوحدة 100 إذن هذه القيمة في هذاالرقم هو وحدة مم همممممم في هذا الرقم على 10 في هذا الرقم 100إذا كان لدينا 10 مرات أكثر وفقًا لذلك ، فسنحصل على رقم إضافي ووزن هذاالرقم سيكون 1000 ومن الواضح من دورة الرياضيات المدرسية أننا نعلم أن 10 من صفرالدرجة تساوي الوحدة 10 في الدرجة الأولى وتساوي 10 10 تربيع 110 الدرجة الثالثةدرجة 1000 ميغاواط نعم لو من نسى هذه المادة سأذكرها بالدرجةيوضح عدد المرات التي يتضاعف فيها الأساس على نفسهمربع أن هذا الأساس يتضاعف مرتين نعم نعم Mhm هنا هما أساساناضرب بعضنا البعض ومن هنا جاءت قيمة 100 لدينا هنا القوة الثلاثةإذن ، 10 يضرب كل منهما الآخر ثلاث مرات 1 10-3 / 10 يضرب كل منهما الآخر وتكون قيمتهماهذا 10 يمكننا تمثيله في صورة 10 مرفوعًا للقوة الأولى وهنا هذا واحدتمثل عطلة نهاية الأسبوع 10 أس صفر يمكننا استخدام هذا الجدول لكتابة القيمة الرقمية لـهذا الرقم لنأخذ رقمًا آخر كمثال لتوضيح ذلك ، دعنا نأخذ الرقم 630 نحناكتب قيمة هذا الرقم ثم ترجمها قلنا لك بالفعل أنه الأدنىالقيمة الرقمية لهذا الرقم مضروبة في 10 درجات هنا نأخذ أقل رقم منقيمة الصفر نضرب في 10 إلى 0 درجة هنا نأخذ الرقم التالي منالقيمة من الرقم ثلاثة التالي نضربه في 10 مرفوعًا للقوة الأولىنعم ثلاثة مضروبًا في 10 مرفوعًا للقوة الأولى ، نأخذ القوة التالية للقوة ستة ونضربها فيأربعة ثم اضرب في 10 إلى الدرجة الثالثة 0123 يومًا بعد التحلل عن طريق حساب قيمة الرقميمكننا أن نرى أن هذه المجموعة من الأرقام لها نفس الشكل ولكن في نظام آخرالحسابات قيمة هذا الرقم ستكون مختلفة تمامًا Uh huh mm هنا مجرد ملفصدفة وماذا سنفعل إذا كان لدينا رقم واحد آخر كيف نغير هذاالصيغة حسناً كالعادة نأخذ هذه القيمة الرقمية ونضع a زائد ونأخذ القيمة مضروبة في 10 وعلى التوالي ، سيكون لدينا 01234 الدرجة الرابعة إذا كانت هناك قيمة أخرى ، قل تسعة زائد 9/10 درجةالعد من الصفر ثم هو صفر رقم أول رقم أول رقم ثاني رقم ثالث رقم رابع رقم خامس نعمنعم مم مم همم على التوالي ودرجة الأفعال الصفرية صفر درجة الرقم الأول وهكذاهذا هو تمثيل قيمة هذا الرقم في النظام العشري شكرا على المشاهدة
 AutoscanOBD ECM PRO ACDEMY
AutoscanOBD ECM PRO ACDEMY
![]()